Team Members: Ryan Collier | Michael Tomaso | Gabriel Esquivel
The project was awarded an honorable mention in the REPEAT competition organized by TEXFAB November 2010.
There is a vast precedent in fabrication projects that deal with the idea of weaving, however within those projects there are more specific techniques. This project concerns itself specifically in the spiral or coil. In "Tooling" Aranda/Lasch describe this technique as, “the spiral (which) is not so much the shape as the evidence of a shape in formation."(1) This idea implies constant movement as a desired effect - something architecture has historically aspired to. Aranda/Lasch continue the argument in terms of spiral lattices as multiple woven points weaving producing stability: this gives the potential of using the technique as away to assure the structure of the project’s form. To employ such a technique, one would have to use materials and tools that provide a ribboning or woven methodology.
When we began to research more about weaving techniques we looked at“coiled” baskets and “plaited” basketry. Baskets are categorized by Technique; we found diagonally plaited as producing a more contemporary effect. At the same time, coiled baskets provide more stability in that they employ a technique
of bundle strands or rods stitched into a spiraling oval or round form with a thin, flexible element to create a coil. Numerous variations of stitch types and embellishments (such as imbrications) can afford a wide range of possibilities.
Spulenkorb is a combination of both techniques. Spulenkorb literally means a coiled or spiral-form basket. The interest in a coil-spiral-weaving technique is the idea of movement - a propelling force that make things operate - a pattern and certain geometries referential to physics and chemistry as well as in popular culture, music, and film - all while being sensitive to architectural logic of elegance in structure and form.
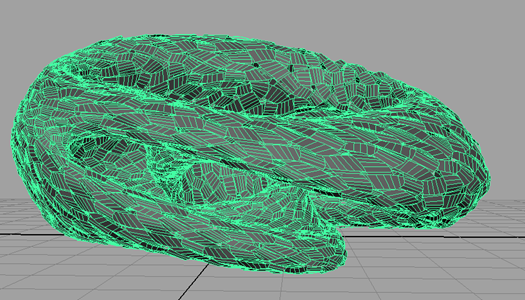
The initial form then was conceived as a mobius rind (2), a variation of the pure mathematical "strip". For initial investigation we worked with the tool TopMod(3), a topological manifold mesh modeler which handles multi-genus geometries, to model the rind. We then continued to develop the mesh in Maya to include vortex apertures and variable, ornamental perforations, in an effort to test therobustness of the weaving algorithm.
Once the basic geometry was developed, the project was then passed through a series of subdivision routines to help determine which might provide the type of weaving that we ultimately desired. The team selected a base cubic pentagonalization routine because of the flower-like aperture treatment and the relationship between the various ribbons. There was also a strong consideration of the necessity of the final algorithm: weaving the mesh required inclusion of mesh based knots and links. These links can be represented in various ways, and can be passed through a subdivision-extrusion-reevaluation procedure to produce the desired woven effect. The final state of the project includes a series of six continuous ribbons of various lengths. Additionally, none of the ribbons are straight, nor do they maintain their width - these variables are determined by the geometry, the algorithm, and the further parametric variables provided in the algorithm. Each ribbon includes several hundred developable surfaces, all with a unique four- sided condition. It is because of these specificities, and because of the recent development of the tools that such a project can be conceived, digitally or otherwise.
In conclusion, that while up to this point much of the study in digital fabrication research has been based on tessellated surfaces typically derived from some abbreviation of the catmull-clark subdivision routine, this proposal supersedes these current studies by providing new solutions and challenges through the use of emerging tools (such as topmod) and algorithims (such as cubic pentagonalization and weaving) providing an opportunity to experiment and new directions.
1. Aranda, Benjamin. Tooling. Aranda/Lasch. Pamphlet Architecture 27. Princeton Architectural Press. Spiraling. P 12.
2. Akleman Ergun. Cyclic Plain Weaving on Polygonal Mesh Surfaces with Graph Rotation Systems. Siggraph 09.
3. see topmod3d.org for more information
Be sure to check out the project on suckerpunch-